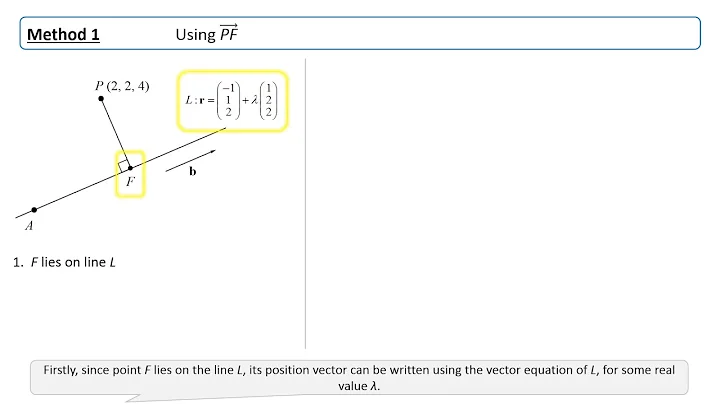
How do you find the foot of a perpendicular point?
Let ax+by+c=0 be a straight line. If a perpendicular line is drawn from any point on the plane to this straight line then the point of intersection of the given straight line and its perpendicular is called the foot of the corresponding perpendicular.
The perpendicular foot, also called the foot of an altitude, is the point on the leg opposite a given vertex of a triangle at which the perpendicular passing through that vertex intersects the side.
For a given line l and point P, if we draw a line through P perpendicular to l, then this line will intersect l at a point Q. The point Q is called the foot of the perpendicular line.
Solution. Let A (−1, 3) be the given point. Lines 3x − 4y − 16 = 0 and AM are perpendicular. Hence, the coordinates of the foot of perpendicular are ( 68 25 , − 49 25 ) .
Since the foot of the perpendicular from the given point will lie on the y-axis, both the x and the y coordinates of the foot of the perpendicular will be equal to zero. Now, since the perpendicular line is normal to the y-axis, it will be parallel to the x-z plane. Therefore, the y-coordinate has to remain constant.
Coordinates and line equation is the prerequisite to finding out the perpendicular line. Consider the equation of the line is ax + by + c = 0 and coordinates are (x1, y1), the slope should be − a/b. If one line is perpendicular to this line, the product of slopes should be -1.
Perpendicular lines are those lines that intersect each other at 90 degrees. Do perpendicular lines have to meet? These lines always intersect at right angles.
Lines that intersect each other forming a right angle are called perpendicular lines. Example: the steps of a straight ladder; the opposite sides of a rectangle.
Perpendicular lines are lines that intersect at a right (90 degrees) angle.
Therefore, the formula for finding the perpendicular distance of a point from a line is: d = | A x 1 + B y 1 + C | A 2 + B 2.
How do you find a perpendicular line between two points?
First, put the equation of the line given into slope-intercept form by solving for y. You get y = -2x +5, so the slope is –2. Perpendicular lines have opposite-reciprocal slopes, so the slope of the line we want to find is 1/2. Plugging in the point given into the equation y = 1/2x + b and solving for b, we get b = 6.
Detailed Solution
Let (h, k) be the coordinates of the foot of the perpendicular from the point (2, 3) on the line 4x - 5y + 8 = 0. Therefore, ( 78 41 , 128 41 ) are the coordinates of the foot of the perpendicular from the point (2, 3) on the line 4x - 5y +8 = 0.
Notice that y = 2x + 3 is in slope-intercept form, and in this equation, m is 2. Thus, the slope of the line y = 2x + 3 is 2. By definition, the slope of any line perpendicular to the line with slope 2 is −12 . Therefore, the slope of any line perpendicular to y = 2x + 3 is −12 .
Now the perpendicular distance of (2,−3) from (0,−3) is =2 units. Was this answer helpful?
In geometry, the perpendicular distance between two objects is the distance from one to the other, measured along a line that is perpendicular to one or both. The distance from a point to a line is the distance to the nearest point on that line.
- In the example problem, multiply like this:
- 5 feet × 12 = 60 inches.
Length between perpendiculars (often abbreviated as p/p, p.p., pp, LPP, LBP or Length BPP) is the length of a ship along the summer load line from the forward surface of the stem, or main bow perpendicular member, to the after surface of the sternpost, or main stern perpendicular member.
180 degrees form a straight line, while 90 degrees form a perpendicular line.
1. The Linear Pair Perpendicular Theorem. The linear pair perpendicular theorem states that when two straight lines intersect at a point and form a linear pair of equal angles, they are perpendicular. A linear pair of angles is such that the sum of angles is 180 degrees.
Any pair of adjacent angles formed by intersecting lines will have a sum of 180 degrees. When the lines are perpendicular, each angle will measure 90 degrees, with a total sum of 180 degrees.
Does perpendicular mean straight?
vertical, perpendicular, plumb mean being at right angles to a base line. vertical suggests a line or direction rising straight upward toward a zenith. the side of the cliff is almost vertical. perpendicular may stress the straightness of a line making a right angle with any other line, not necessarily a horizontal one ...
In geometry a perpendicular angle is 90 degrees, a perfect L. On a compass, East and North are perpendicular to each other. The term can be used more generally to describe any steep angle.
at an angle of 90° to another line or surface: Two perpendicular lines form a right angle. Perpendicular is also standing or rising straight up: The cliff was nearly perpendicular and impossible to climb.
Since x=8 is a vertical line, the slope is undefined. The perpendicular line is horizontal and the slope is 0 .
270 Degree Angle
This angle is formed by perpendicular lines like for a 90 degree angle, but in this case the angle being measured is the other side of the right angle. This is an example of a "reflex angle," which is any angle that measures between 180 and 360 degrees.
A line perpendicular to a horizontal line is a vertical line. A vertical line, by definition has a slope which is undefined. Therefore the slope of any line perpendicular to y=10 is undefined.
As, perpendicular is drawn from point P to y-axis, so distance of point of intersection of this line from x z plane remains the same. ∴ Length of foot of perpendicular drawn from the point P (3, 4, 5) on y-axis is √34 units.
The vector equation of the plane passing through A and perpendicular to n is r n a n. n ¯ = a ¯ . n ¯ . M(1,0,0) is the foot of the perpendicular drawn from origin to the plane.
L is the foot of the perpendicular drawn from a point P (3, 4, 5) on the xy-plane. The coordinates of point L are. As L lies on xy plane, so its distance from xy plane is zero or we can say that z-coordinate is 0.
To find a line that's perpendicular to a line and goes through a particular point, use the point's coordinates for (x1, y1) in point slope form: y - y1 = m (x - x1). Then, calculate the "negative reciprocal" of the old line's slope and plug it in for m.
What is the distance between two perpendicular lines?
The distance is the perpendicular distance from any point on one line to the other line. The shortest distance between such lines is eventually zero. The distance is equal to the length of the perpendicular between the lines.
Distance between two parallel lines is given by the formula d = |c1-c2|/√(a2 + b2).
The length of perpendicular drawn from the point (4, 5) to the line 3x + 4y = 10 is: 42/5.
The perpendicular distance of point (4,3) from X−axis=3 units. Was this answer helpful?
Since L is the foot of perpendicular from P on the xy-plane z-coordinate is zero in the xy-plane. Hence coordinates of L are 6 7 0.
- Step 1: Determine the equation of the Line. Let A = ( cos θ , sin θ ) and B = ( cos ϕ , sin ϕ ) be the given points. Then equation of is of the form, ...
- Step 2: Determine the distance. We know that distance of this line from the origin, D = 0 - 0 + sin ( θ - ϕ ) ( sin ϕ - sin θ ) 2 + ( cos ϕ - cos θ ) 2.
- A. y = 0.
- B. x = 0.
- C. a = – a.
- D. y = – a.
We know that the foot of the perpendicular from focus to any tangent of parabola lies on the tangent at the vertex of that parabola and it is the midpoint of the line segment joining the focus and the point on the directrix.
So, the answer is 3 units.
The perpendicular distance of the point P (4,3) from y-axis is 4.